Quantum Gates: Single Qubit Gates: Quantum Not Gate, Pauli – X, Y And Z Gates, Hadamard Gate, Phase Presentation

Introduction to Quantum Gates
Quantum gates are fundamental building blocks in quantum computing. Single qubit gates operate on a single qubit, modifying its state. In this presentation, we will explore several important single qubit gates.	Image Source
		1

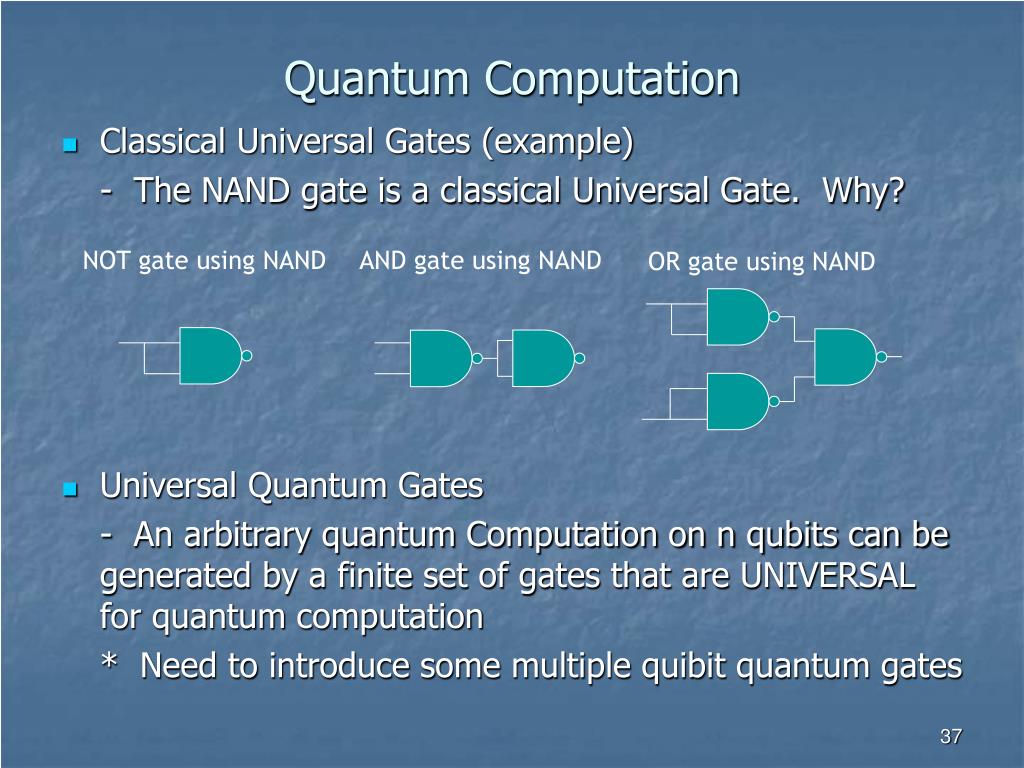
Quantum Not Gate
The Quantum Not gate is analogous to the classical NOT gate. It flips the state of a qubit, mapping 0 to 1 and 1 to 0. The matrix representation of the Quantum Not gate is [0 1, 1 0].
		2

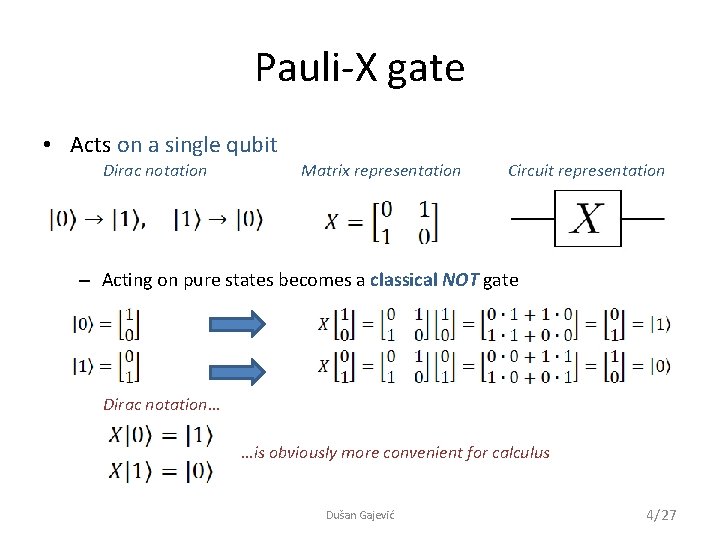
Pauli-X Gate
The Pauli-X gate is another name for the Quantum Not gate. It is represented by the matrix [0 1, 1 0]. The Pauli-X gate flips the state of a qubit along the X-axis of the Bloch sphere.	Image Source
		3

Pauli-Y Gate
The Pauli-Y gate is represented by the matrix [0 -i, i 0]. It flips the state of a qubit along the Y-axis of the Bloch sphere. The Pauli-Y gate introduces a phase shift of π/ 2.	Image Source
		4

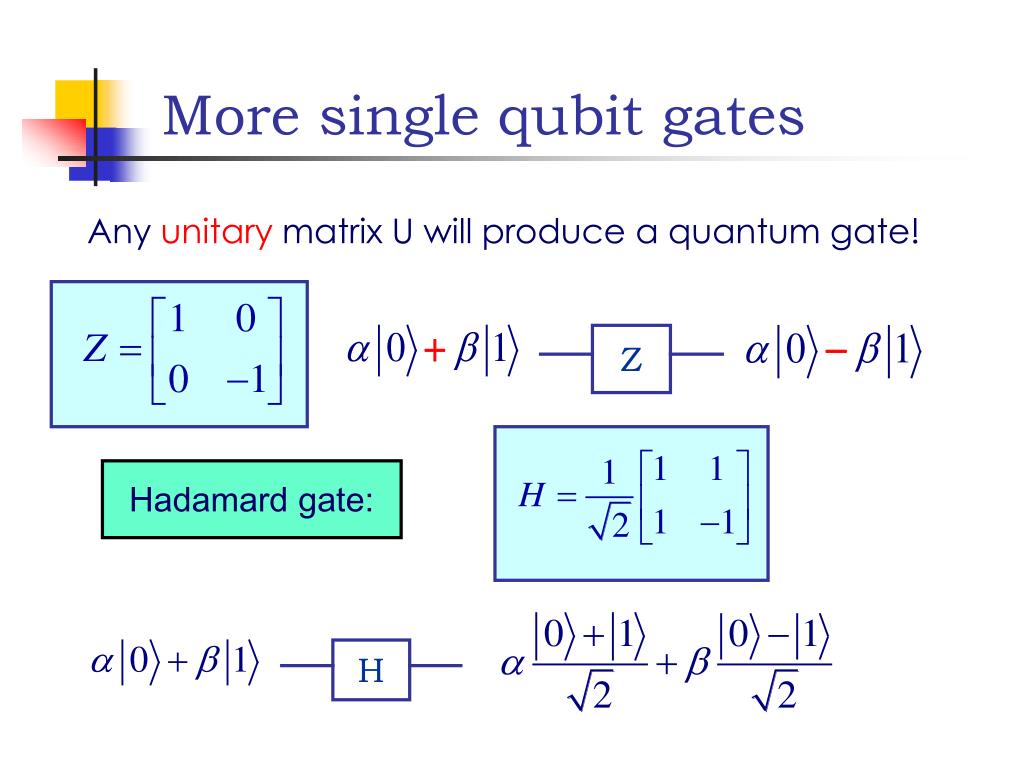
Pauli-Z Gate
The Pauli-Z gate is represented by the matrix [1 0, 0 -1]. It flips the sign of the phase of a qubit. The Pauli-Z gate leaves the state of the qubit along the X and Y axes unchanged.	Image Source
		5

Hadamard Gate
The Hadamard gate is represented by the matrix [1 1, 1 -1]/ √2. It creates superposition by transforming the basis states. The Hadamard gate maps |0⟩ to (|0⟩ + |1⟩)/ √2 and |1⟩ to (|0⟩ - |1⟩)/ √2.
		6

Phase Gate
The Phase gate is represented by the matrix [1 0, 0 e^(iθ)]. It introduces a phase shift of θ to the state of a qubit. The Phase gate does not change the probability amplitudes of the basis states.	Image Source
		7

Summary
Quantum gates are essential for manipulating qubit states. Quantum Not, Pauli-X, Pauli-Y, and Pauli-Z gates perform state flips. The Hadamard gate creates superposition, while the Phase gate introduces phase shifts.	Image Source
		8

Applications
Single qubit gates are used in various quantum algorithms. Quantum error correction relies on gate operations for error detection and correction. Single qubit gates are crucial for implementing quantum teleportation and quantum cryptography.	Image Source
		9

Conclusion
Single qubit gates play a vital role in quantum computing. Quantum Not, Pauli-X, Pauli-Y, Pauli-Z, Hadamard, and Phase gates are key examples. Understanding and utilizing these gates is essential for harnessing the power of quantum computing.	Image Source
		10

References (download PPTX file for details)
Nielsen, M. A., & Chuang, I. L. (2010). Quant... Your second bullet... Your third bullet...
		11

Join the SlideMake Community