Laplace Transform Of Periodic Function Presentation

Introduction to Laplace Transform of Periodic Function
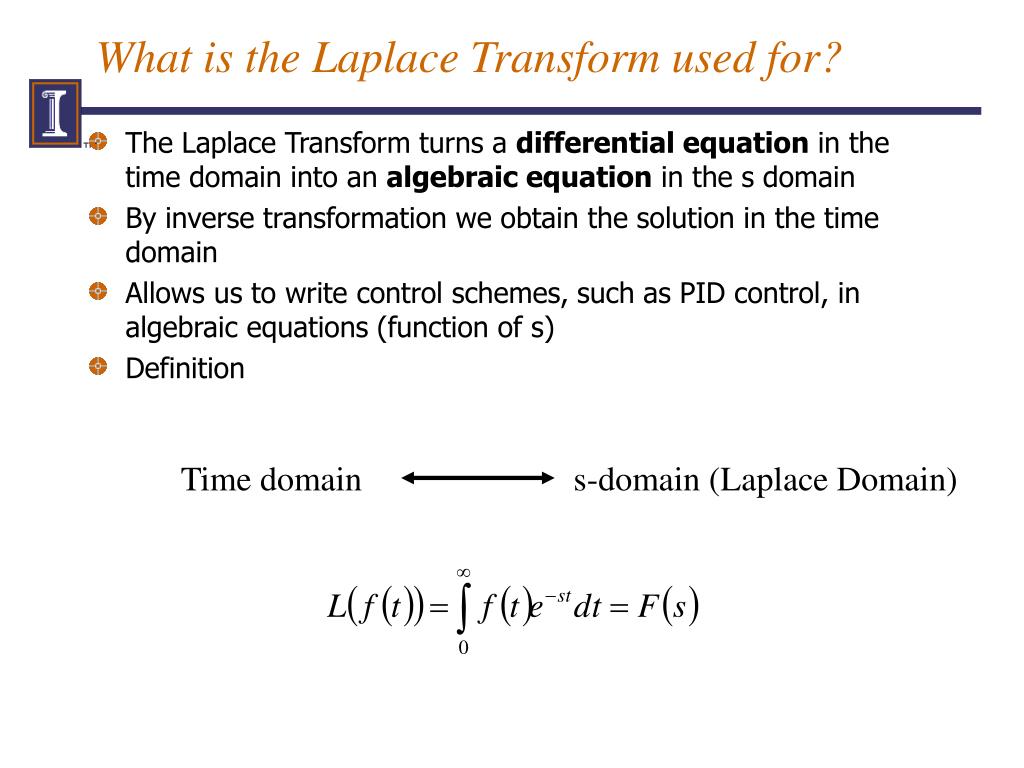
The Laplace transform is a mathematical technique used to transform a function of time into a function of complex frequency. A periodic function is a function that repeats itself in regular intervals. The Laplace transform of a periodic function allows us to analyze its behavior in the frequency domain.	Image Source
		1

Definition of Laplace Transform of Periodic Function
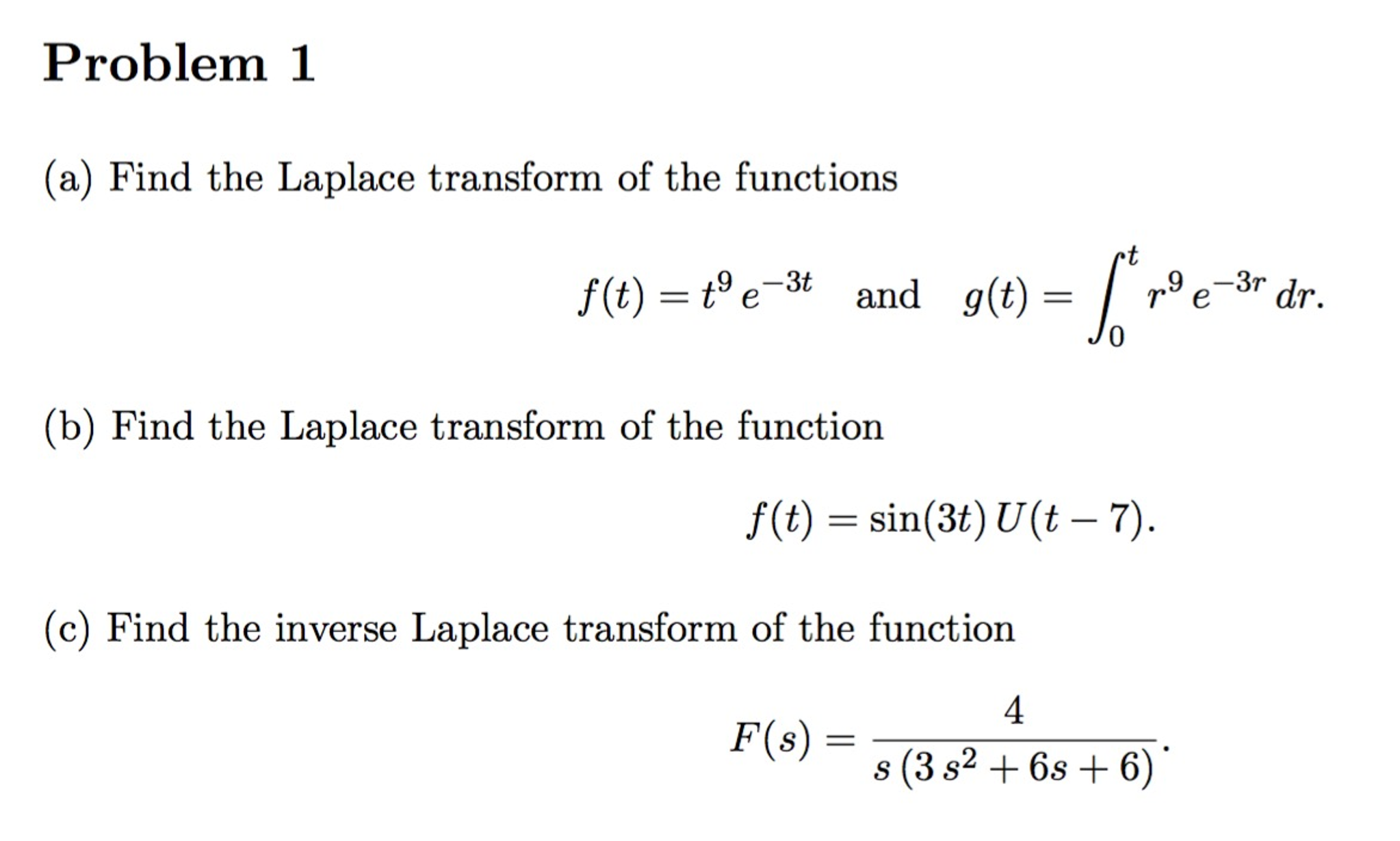
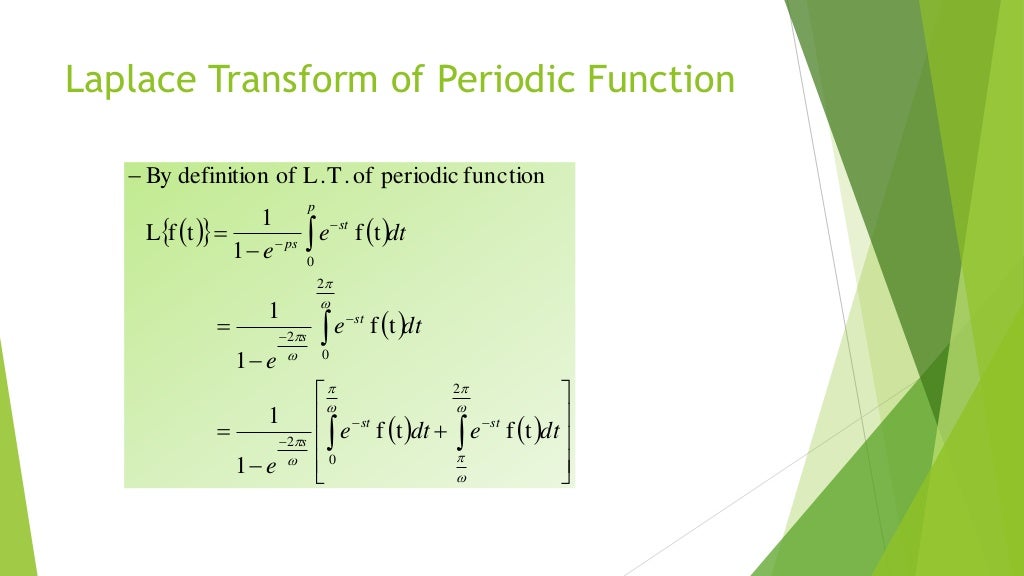
The Laplace transform of a periodic function f(t) is given by the formula: F(s) = (1 - e^(-Ts)) / (s - jω), where s is the complex frequency. T is the period of the periodic function, and ω is the angular frequency. The Laplace transform of a periodic function exists only if the function satisfies certain conditions, such as being piecewise continuous and having a finite number of maxima and minima within each period.	Image Source
		2

Properties of Laplace Transform of Periodic Function
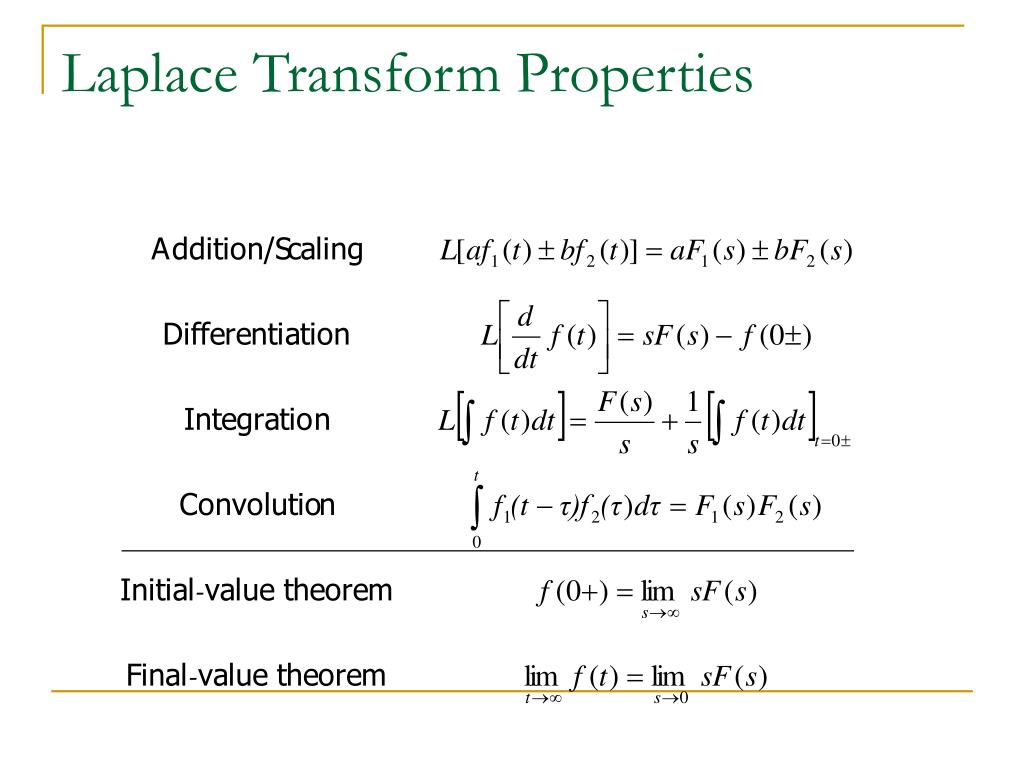
The Laplace transform of a periodic function can be used to find its frequency spectrum, which indicates the presence and strength of different frequencies. The Laplace transform of a periodic function is a rational function in the complex frequency domain, allowing us to analyze its poles and zeros. The Laplace transform of a periodic function can be used to solve differential equations involving periodic functions.	Image Source
		3

Example of Laplace Transform of Periodic Function
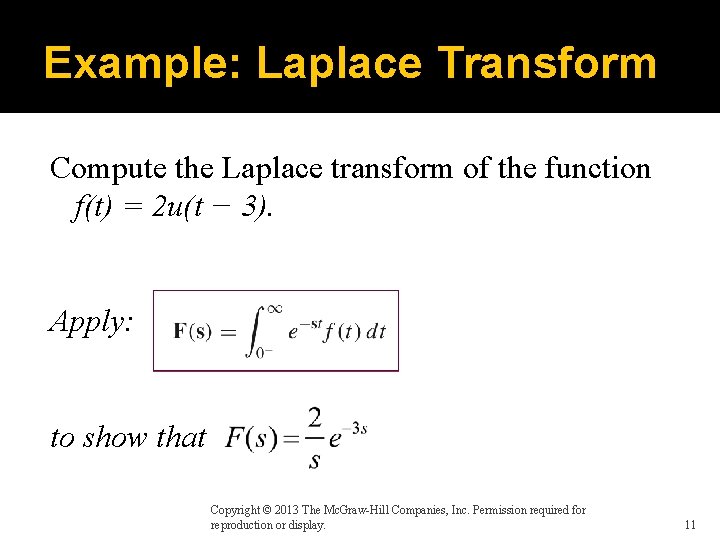
Let's consider a periodic square wave function with a period T and amplitude A. The Laplace transform of the square wave function is given by F(s) = (A/ T) (1 - e^(-Ts)) / (s - jω), where ω = 2π/ T.	Image Source
		4

Applications of Laplace Transform of Periodic Function
The Laplace transform of a periodic function is widely used in electrical engineering to analyze and design circuits with periodic signals. It is used in control systems to analyze the stability and response of periodic systems. The Laplace transform of a periodic function is also used in signal processing to analyze and manipulate periodic signals.	Image Source
		5

Limitations and Challenges of Laplace Transform of Periodic Function
The Laplace transform of a periodic function assumes that the function is defined for all time, which may not be the case in practical applications. The Laplace transform may not exist for certain periodic functions that do not satisfy the required conditions. The Laplace transform of a periodic function can sometimes be challenging to calculate analytically, requiring numerical methods or approximation techniques.	Image Source
		6

Conclusion
The Laplace transform of a periodic function provides a powerful mathematical tool for analyzing periodic signals in the frequency domain. It allows us to understand the frequency content, stability, and response of periodic systems. Despite its limitations and challenges, the Laplace transform of a periodic function is widely used in various fields of science and engineering.	Image Source
		7

Join the SlideMake Community