Applications Of Differential Calculus Presentation

Introduction to Applications of Differential Calculus
Differential calculus is a branch of mathematics that studies the rate at which quantities change. It is widely used in various fields to model and solve real-world problems. Applications of differential calculus range from physics and engineering to economics and biology.	Image Source
		1

Optimization Problems
Differential calculus helps in solving optimization problems, where we aim to find the maximum or minimum value of a function. It is used in engineering to optimize the design of structures, such as bridges or buildings, by minimizing costs or maximizing strength. In economics, it can be applied to maximize profits or minimize costs for businesses.	Image Source
		2

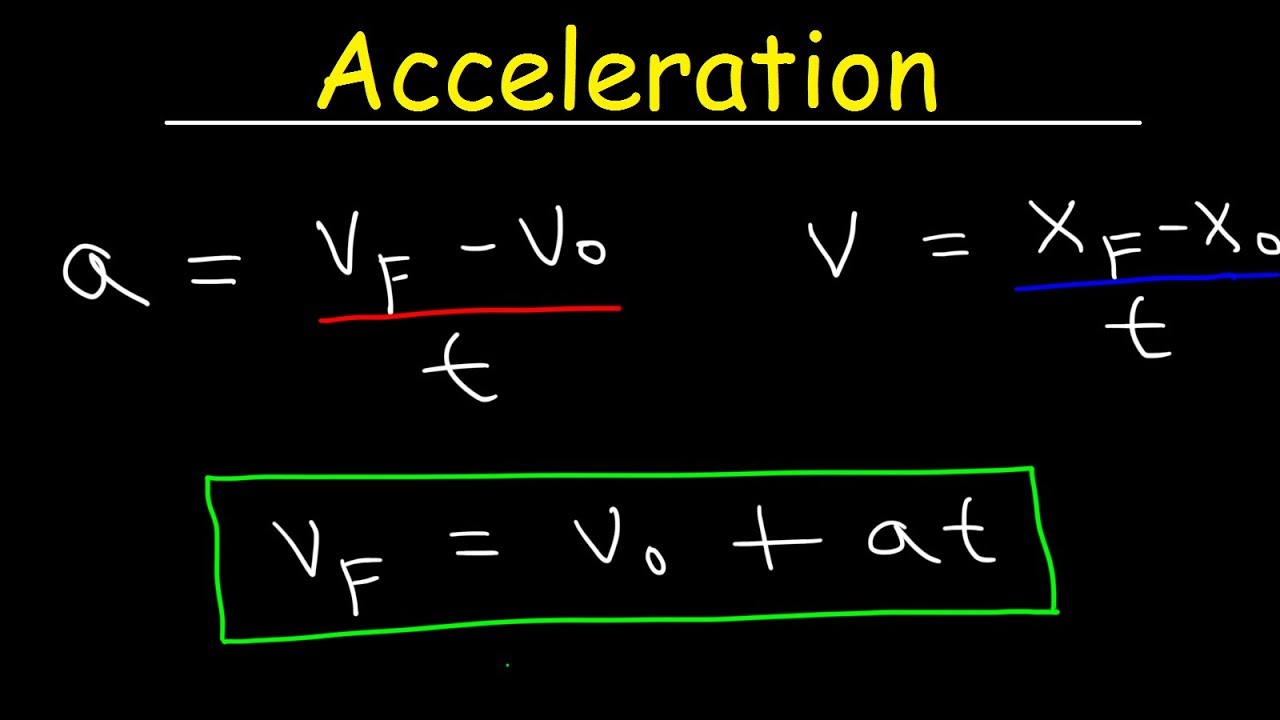
Calculating Rates of Change
Differential calculus allows us to calculate rates of change, such as velocity or acceleration, in physics and engineering. It is used in astronomy to determine the rate at which a star is moving away from us based on its redshift. In biology, it helps model population growth rates or the rate of change in enzyme activity.	Image Source
		3

Curve Sketching
Differential calculus helps us analyze and sketch the behavior of functions. It helps determine the critical points, inflection points, and concavity of a function. Curve sketching is essential in physics, engineering, and economics to understand the behavior of variables.	Image Source
		4

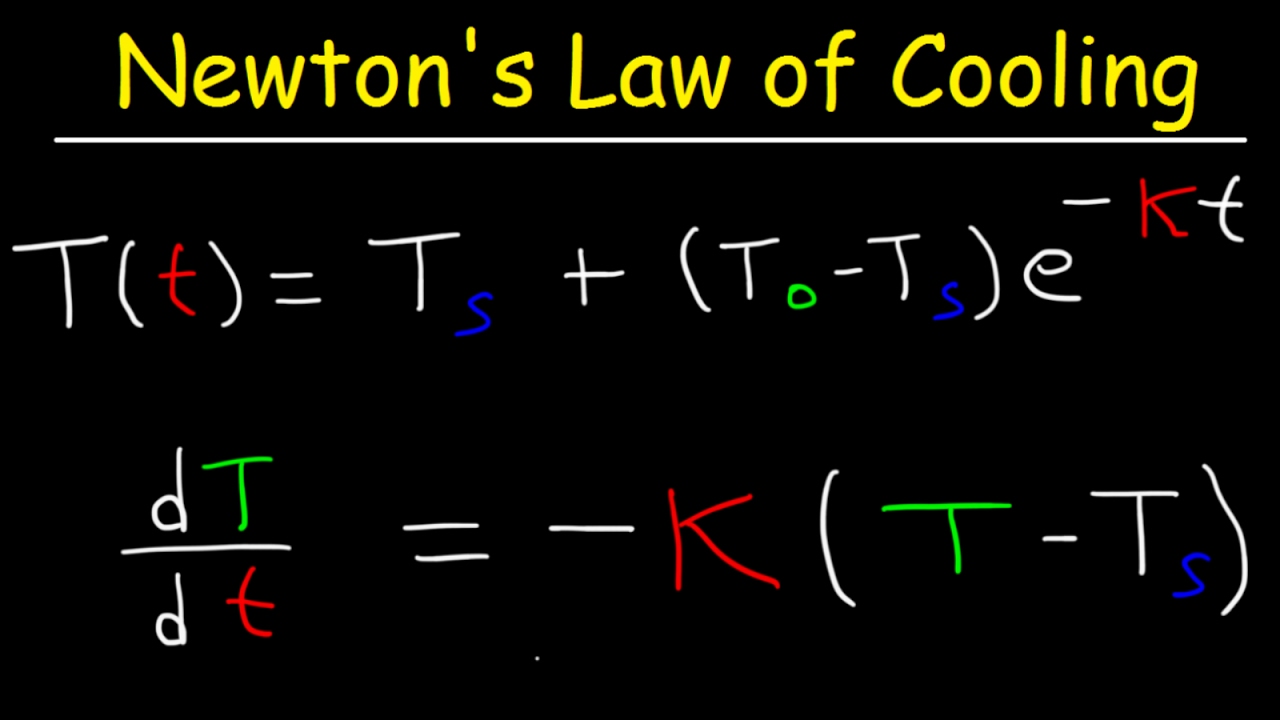
Newton's Law of Cooling
Differential calculus is used to model the cooling of an object over time. It helps determine the rate at which the temperature of an object changes. Newton's Law of Cooling finds applications in engineering, meteorology, and food science.	Image Source
		5

Related Rates
Differential calculus is applied to solve problems involving related rates, where two or more variables are changing with respect to time or another variable. It is used in physics to analyze the motion of objects in relation to each other. Related rates problems also find applications in navigation, engineering, and medical imaging.	Image Source
		6

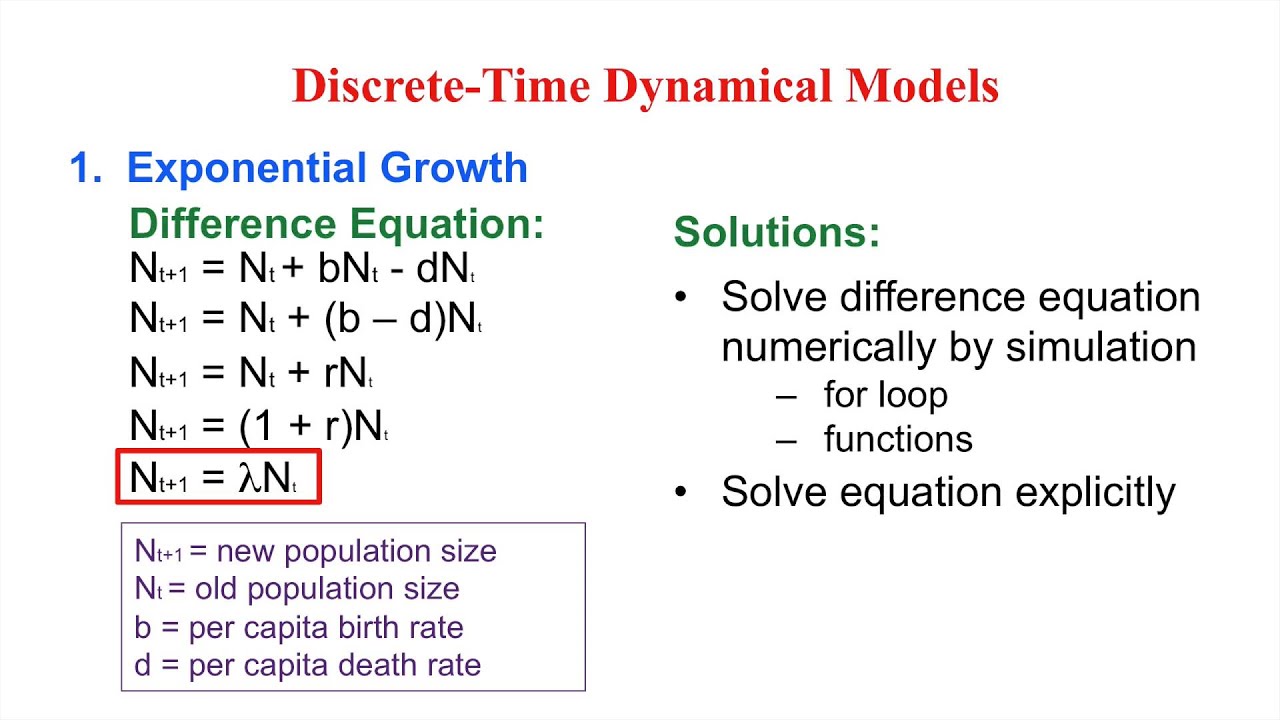
Population Dynamics
Differential calculus is used to model and analyze population dynamics. It helps determine the rate of change of a population with respect to time. Population dynamics is crucial in ecology, epidemiology, and urban planning.	Image Source
		7

Economic Analysis
Differential calculus is employed in economic analysis to study supply and demand functions. It helps determine the elasticity of demand or supply, which measures the responsiveness of quantity demanded or supplied to changes in price. Economic analysis using calculus is vital for policy-making and understanding market behavior.	Image Source
		8

Fluid Dynamics
Differential calculus is used extensively in fluid dynamics to model and analyze the behavior of fluids. It helps determine fluid flow rates, pressure gradients, and fluid forces. Applications of fluid dynamics range from designing efficient pipelines to understanding weather patterns.	Image Source
		9

Conclusion
Differential calculus plays a vital role in various fields, enabling us to understand and solve complex real-world problems. Its applications span physics, engineering, economics, biology, and many other disciplines. The versatility of differential calculus makes it a powerful tool for modeling and analyzing change and optimization problems.	Image Source
		10

References (download PPTX file for details)
Stewart, J. (2015). Calculus: Early Transcend... Anton, H., Bivens, I., & Davis, S. (2013). Ca... Larson, R., Edwards, B., & Hostetler, R. (201...
		11

Join the SlideMake Community